MA441- LEC 1
Goal
- Understanding the movitation of calculus
- Understanding the tangent line of a curve.
- average and instantaneous velocity
Two strategies in mathematics/computer science
Divide and conquer
- Break the problem into sub-problems
- Solve the trivial cases
- Combine sub-problems to the original problem.
Reduction Method
Assume problem A is reducible to an easy problem B. If we can solve problem B, then we can solve problem A.
Why Calculus?
- Area problem
- Tangent line
Area problem I
Approximating Area of a Circle
Area problem II
Approximating area of a function
tangent line
"Tangent" means touching. So tangent line to a curve is a line that touches the curve.
The Secant line \(\overline{PQ}\) is the line passing through point \(P\) and \(Q\).
How can you find the slope of the secant line \(\overline{PQ}\), \(m_{PQ}\)?
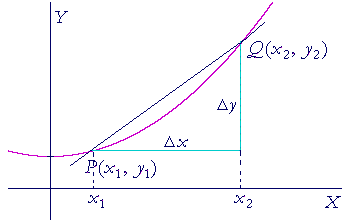
\[m_{PQ}=\frac{y_2-y_1}{x_2-x_1}\]
How can you calculate the slope of tangent line at a point \(P\)?
Find the slope of tangent line of \(f(x)=\frac{x^2}{4}\) at \(x=2\).
From above experiment, what do you expect?
Physics
Let \(y=f(x)\) be a position function of a car at time \(x\).
\[ average~velocity=\frac{\Delta distance}{\Delta time}=\frac{\Delta y}{\Delta x} \]
which is the slope of secant line.
How about an instantaneous velocity at time \(x\)?
\[ instantaneous~velocity =?=slope~of~tangent~line \]
History
Newton

Born: 25 December 1642
Principia Mathematica(1687)
